Draw Axes of a 2D Covariance Ellipse

A function to draw the principal axes of a 2D ellipse from a correlation, covariance or sums of squares and cross products matrix in an existing plot.

Usage

ellipse.axes(
  x,
  centre = c(0, 0),
  center = centre,
  scale,
  which = 1:2,
  level = 0.95,
  radius = sqrt(qchisq(level, 2)),
  labels = TRUE,
  label.ends = c(2, 4),
  label.pos = c(2, 4, 1, 3),
  type = c("lines", "arrows"),
  ...
)

Arguments

x

A square positive definite matrix at least 2x2 in size. It will be treated as the correlation or covariance of a multivariate normal distribution.

centre, center

The center of the ellipse

scale

If x is a correlation matrix, then the standard deviations of each parameter can be given in the scale parameter. This defaults to c(1, 1), so no rescaling will be done.

which

An integer vector to select which variables from the object x will be plotted. The default is the first 2.

level

The coverage level of a simultaneous region of the ellipse. The default is 0.95, for a 95% region. This is used to control the size of the ellipse.

radius

The size of the ellipsoid may also be controlled by specifying the value of a t-statistic on its boundary. This defaults to the square root of a chi-square statistic for a given level on 2 degrees of freedom, however in a small sample of n observations, a more accurate value is sqrt(2 * qf(level, 2, n - 1 )).

labels

Either a logical value, a character string, or a character vector of length 2. If TRUE, the default, the axes are labeled "PC1", "PC2". If a single character string, the digits 1, and 2 are pasted on the end.

label.ends

A vector of indices in the range 1:4 indicating which ends of the axes should be labeled, corresponding to a selection of rows of the 4 x 2 matrix of axes end points. Values 1:2 represent the minimum and maximum of the first dimension respectively. Values 3:4 represent the minimum and maximum of the second dimension. Default: c(2, 4).

label.pos

Positions of text labels relative to the ends of the axes used in text for the four possible label.ends. 1, 2, 3, 4 represent below, to the left, above and to the right. The default, c(2, 4, 1, 3), positions the labels outside the axes.

type

Character. Draw "lines" or "arrows".

...

Other arguments passed to lines and text.

Value

Invisibly returns a 4 x 2 matrix containing the end points of the axes in pairs (min, max) by rows.

See also

lines, text

Author

Michael Friendly

Examples

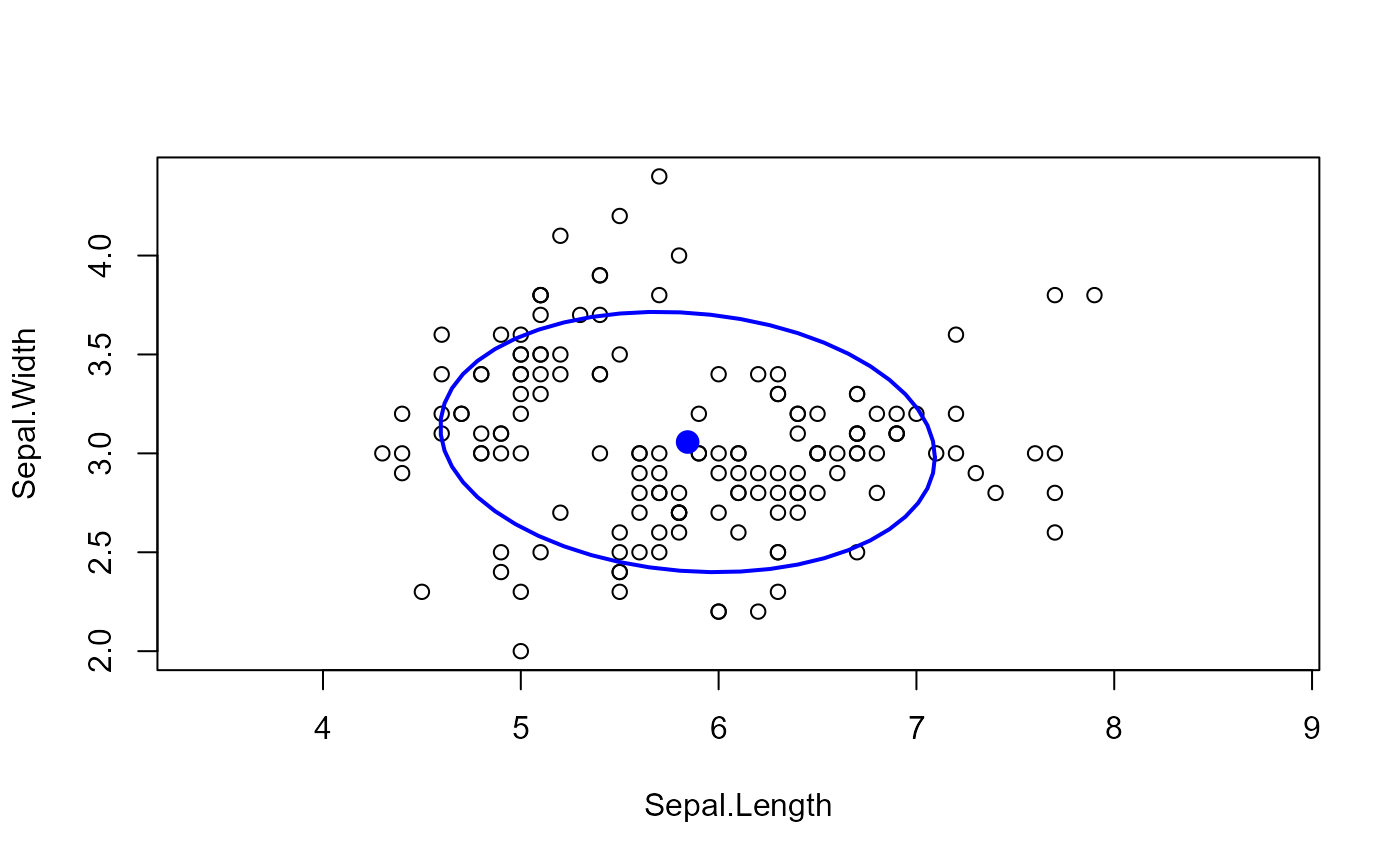
data(iris)
cov <- cov(iris[,1:2])
mu <- colMeans(iris[,1:2])

radius <- sqrt(qchisq(0.68, 2))
plot(iris[,1:2], asp=1)
car::ellipse(mu, cov, radius = radius)
res <- ellipse.axes(cov, center=mu, level = 0.68,
                    labels = TRUE)

res
#>      Sepal.Length Sepal.Width
#> Xmin     4.594510    3.163463
#> Xmax     7.092157    2.951204
#> Ymin     5.788148    2.407969
#> Ymax     5.898519    3.706698

# try some options 
plot(iris[,1:2], asp=1)
car::ellipse(mu, cov, radius = radius)
abline(h=mu[2], v=mu[1], col = "grey")
ellipse.axes(cov, centre=mu, level = 0.68,
             labels = "Dim", label.ends = 1:4,
             lwd = 2, lty = 2, col = "red",
             cex = 1.5)

             
# draw arrows rather than lines            
plot(iris[,1:2], asp=1)
car::ellipse(mu, cov, radius = radius)
ellipse.axes(cov, center=mu, level = 0.68,
             type = "arrows")